A VI teacher writes:
I have a seventh grade braille student who will soon be studying a math chapter in a regular classroom. Among the topics are the following:
- Translations (slides)
- Reflections
- Line Symmetry
- Tessellations
I have some ideas for the teacher. However, being blind myself, I know these concepts can be very difficult to grasp. I would appreciate any ideas which I might share with the classroom teacher.
Susan replies:
I usually introduce translations, reflections, and rotations (sometimes called transformations) together. As a firm believer in the use of manipulatives (for the sighted as well as the blind), I pull out my box of assorted triangles and quadrilaterals. I select two congruent non-regular polygons and place one on top of the other; two scalene triangles are my favorite. I then proceed to slide, flip, or rotate the top manipulative to demonstrate a translation, reflection, or rotation. The bottom manipulative remains in place as the original figure. This correlates well with most print textbooks which may show the original figure in red and the transformed figure in black. If you wish the student to translate a figure to a given point, rotate it to a new position, and reflect it over a given line, you could use four congruent figures. I would probably want to use magnetic manipulatives or ones with Velcro in a confined space, to keep things in place. Be sure to show the student the textbook tactile graphics illustrating the same transformations, so they will become familiar with what the “average” textbook furnishes them. If these graphics are not of high quality, make your own using some type of Stereocopier and capsule/swell paper. Furthermore, I show my students examples of test questions on transformations from one of the many standardized mathematics release tests in braille.
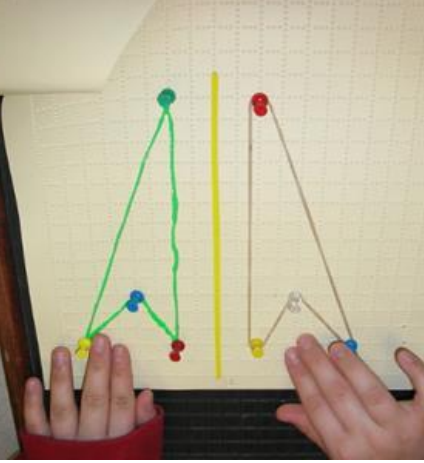
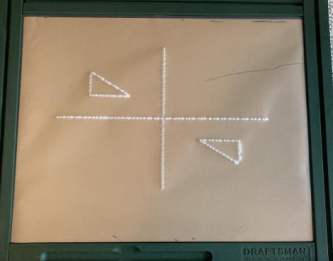
When we reach the topic of line symmetry, I remind my students of when they were younger and made valentine hearts by cutting a folded piece of paper. Believe it or not, my high school students have fun folding a piece of braille paper and cutting out hearts or some other symmetrical design. I tell them the folded edge is a line of symmetry. Then, I get out my manipulative box again, selecting two congruent right triangles. After placing one on top of the other, I flip (reflect) the one on top over the line segment formed by one of the legs to create a larger isosceles triangle with a line of symmetry (altitude) down the middle. You can also have your student use paper folding to determine symmetry lines for figures studied so far (rectangles, hexagons, etc.). Again, be sure to show the student the textbook tactile illustrations of symmetry and/or make your own graphics as outlined above.

Tessellations or tiling patterns is an arrangement of figures that fill a plane but do not overlap or leave gaps. In a pure tessellation, the same figure is used throughout. I usually begin with having my students check out my classroom floor, which is composed of square tiles. I also have a set of tables in the shape of isosceles trapezoids, which create a tessellation. Then I move to textbook or home-made tactile graphics of tessellations using rectangles, equilateral triangles, parallelograms, right triangles, regular hexagons, etc. Let the students explore to find that any triangle or quadrilateral can be used to tessellate a plane, but that only certain polygons with more than four sides tessellate a plane. Tessellations that use more than one type of polygon are called semi-pure tessellations. At this point, I get out my wooden Discovery Blocks from ETA (various and duplicate sizes of triangles, squares, rectangles, and parallelograms) and let them design their own tessellation. One young man designed an incredibly beautiful tessellation and placed the blocks inside a frame. It was quite a magnificent piece of parquetry.

Be sure and allow your students to use their imaginations to draw and create their own transformations, line symmetry, and tessellations using paper folding and scissors, 2D manipulatives, the APH Graphic Aid for Mathematics or Graph Paper with push pins and rubber bands, geometry stencils (templates), the APH Draftsman or TactileDoodle, and any other combination they can concoct.
This article was originally published by Texas School for the Blind and Visually Impaired (TSBVI) and is reprinted here with permission. Updated in 2024.