A VI teacher inquires
My first love is braille, but I am being challenged this year, as it is my first year in over 16 years that I am co-teaching Pre-Algebra to a blind student. Next week it is factor trees; I can solve that one okay but I won’t ignore any suggestions either.
Susan replies
Now that scientific graphing calculators are required for so much in the secondary mathematics curriculum, I am happy to suggest one kind of neat use for the abacus. My students use the “Osterhaus” method for prime factorization on the abacus. They tend to cringe at “factor trees;” however, I know that’s the way they teach it in Pre-Algebra, or at least introduce it. Eventually, however (in my book at least) they show them the “repeated division” method. The Osterhaus method is simply this repeated division method done on the abacus. I usually just show people how to do it, and it’s difficult to put it all into words. Nevertheless, I’ll try.
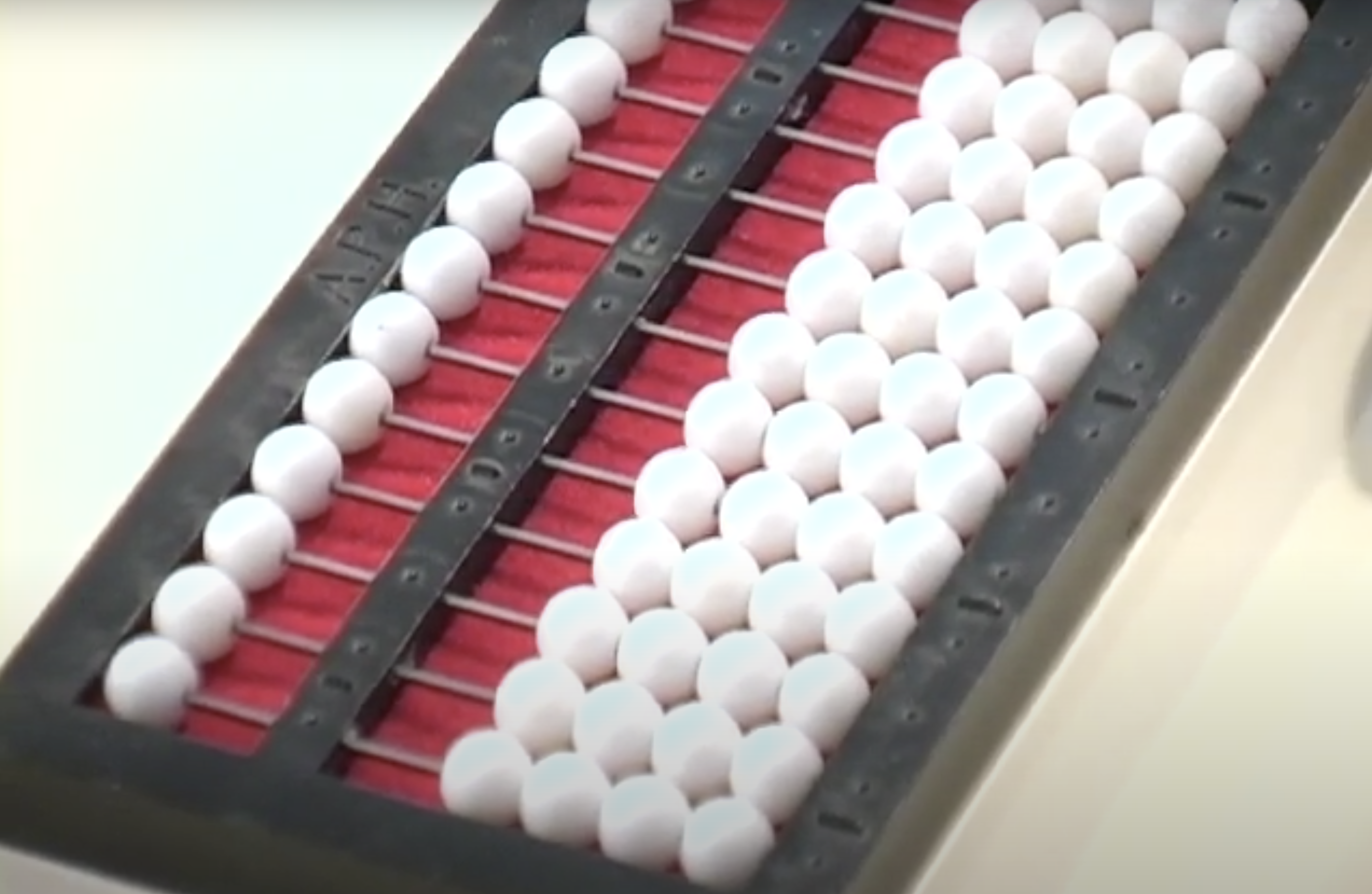
Place the whole number to be factored at the extreme right of the abacus; we’ll call this the dividend. Then, beginning with 2 (the smallest prime number), check whether each prime is a factor of the dividend. If it is, place this first prime number on the extreme left of the abacus and divide the dividend by the prime. Replace the dividend with your answer (quotient) which becomes your new dividend. Continue (putting each new prime that is a factor in the column to the right of the last factor and replacing the quotient with a new dividend) until you arrive at a quotient of 1.
For example, set the whole number 420 at the extreme right of the abacus (4 in the hundreds, 2 in the tens, and 0 in the ones column). Beginning with 2, you find that it is a factor of 420. Therefore, you place 2 on the extreme left of the abacus (trillions column) and replace 420 with 210 at the extreme right of the abacus. You try 2 again and find it is a factor of 210. Place another 2 directly to the right of the first 2 (hundred billions column) and replace 210 with 105 at the extreme right. 2 is not a factor of the new dividend, so you try 3. 3 is a factor of 105. Place 3 directly to the right of the 2nd 2 (10 billions column) and replace 105 with 35 at the extreme right. Is 3 a factor of 35? No, so we try the next prime of 5. Yes, 5 is a factor of 35. Therefore, we place 5 directly to the right of the 3 on the left of the abacus (billions column) and replace 35 with 7 at the extreme right. 5 is not a factor of 7, but 7 is. Therefore, place 7 directly to the right of the five on the left of the abacus (hundred millions column) and replace 7 with 1 at the extreme right. Since your quotient is now 1, you have completed the prime factorization. Reading from left to right, your factorization would be: 2 x2 x 3 x5 x 7 or 22 x3x5x7.
You could have all kinds of variations. If the number to be prime factored is quite large, you may wish to use two abaci – one for the dividend and one for the factors. Some students may need to have a space between factors, which again might require two abaci. If the number is extremely large, the student may wish to use a calculator for the repeated divisions and an abacus (abaci) for recording the factors. My students use this prime factorization method when they need to determine the Greatest Common Factor (GCF) or Least Common Multiple (LCM) for rather large numbers.
See also three prime factorization videos.
This article was originally published by Texas School for the Blind and Visually Impaired (TSBVI) and is reprinted here with permission. Updated in 2024.